B) MELODIC MINOR SCALE
The melodic minor scale differs from the natural minor scale in that it has a raised 6th and a raised 7th note. So the step pattern is therefore:
| 1st | to | 2nd | = 1 tone | (2 frets) |
| 2nd | to | 3rd | = 1 semitone | (1 fret) |
| 3rd | to | 4th | = 1 tone | (2 frets) |
| 4th | to | 5th | = 1 tone | (2 frets) |
| 5th | to | 6th | = 1 tone | (2 frets) |
| 6th | to | 7th | = 1 tone | (2 frets) |
| 7th | to | octave | = 1 semitone | (1 fret) |
So, in the key of A, we get:
| tone | semitone | tone | tone | tone | (#6th) | semitone | (#7th) | tone | |||||||
| A | ^ | B | ^ | C | ^ | D | ^ | E | ^ | F# | ^ | G# | ^ | A | |
| Frets: | 2 | 1 | 2 | 2 | 2 | 2 | 1 |
On the guitar, we could play it on the G string (3rd string) like this:
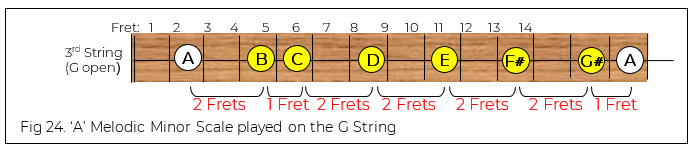
Listen:
Since it is not entirely functional to play a scale on a single string, we can find the notes of this scale across all strings, as outlined below.
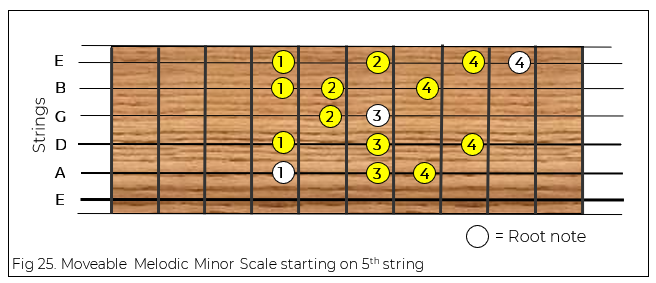
FINGERING FOR ROOT NOTE ON 5TH STRING:
This pattern can be used when the root note of the natural minor scale falls anywhere on the 5th string.
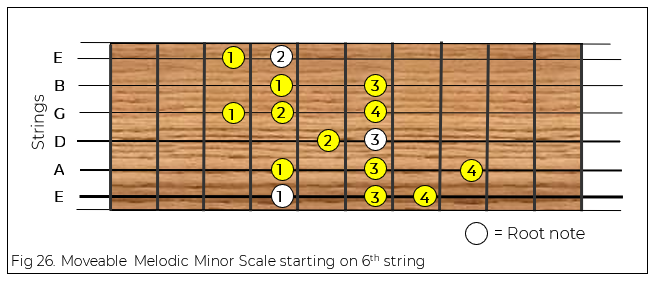
FINGERING FOR ROOT NOTE ON 6TH STRING:
This pattern can be used when the root note of the natural minor scale falls anywhere on the 6th string.